The Difference Quotient Calculator is a free online calculator. Moreover, a difference quotient calculator online can help you calculate the difference quotient for a given function. Then, this difference of quotient calculator performs stepwise calculations to determine the slope of a secant line that connects two locations. In this article, we are talking about this calculator. So, please keep reading to know more about it.

Difference quotient calculator definition
The difference quotient is used in Calculus to compute the slope of the secant line between two points. The slope is significant in calculus since it defines a function’s derivative. Assuming A and B are two points on the graph function f(x), the formula for calculating the slope of the secant line “m” is: m= [f(x+h)-f(x)]/h
This approach is Newton’s Quotient or Fermat’s Difference Quotient.
Difference quotient calculator formula
The difference quotient is used in single-variable calculus to compute the slope for the secant line (i.e., the line that passes between two points on a curve) between any two points on a graph for the function f. The difference quotient for a function f (x) is f(x+h) – f(x) / h, where h is the point difference and f(x+h) – f(x) is the function difference. The slope of curved lines may be determined using the difference quotient formula. When working with curved lines, the f(x+h) – f(x) / h calculator may be used to obtain the slope value. Please feel free to use our online difference quotient calculator to calculate the difference quotient by entering an equation.
The following are the methods we use to determine the difference quotient:
f(x+h)-f(x)/h
- Insert x + h into the function f and simplify to get f(x + h).
- Now that you’ve calculated f(x + h), calculate f(x + h) – f(x) by inserting in f(x + h) and f(x) and simplifying.
- Fill in your step 2 result for the numerator in the difference quotient and simplify it.
This, when pushed to its logical conclusion as h approaches zero, yields the derivative of the function f The expression gets its name from the fact that it is the quotient of the function’s difference in values by the difference in the corresponding values of its input (in this example, (x+h)-x=h).
Difference quotient calculator with steps
Please follow the instructions below to obtain the difference quotient of the given function using the online difference quotient calculator:
- First, navigate to Cuemath’s online difference quotient calculator.
- Then, enter the function into the difference quotient calculator’s supplied input box.
- Then, press the “Calculate” button to compute the difference quotient of the specified function.
- Atlast, click the “Reset” button to clear the box and input new values.
Difference quotient calculator with h
Assume we have a function f. (x). Assume we have a secant line that intersects the curve at two locations (x, f(x)) and (x + h, f(x + h)). The slope of the secant line is then calculated as follows:
Slope = [ f(x + h) – f(x) ] / [ (x + h) – x]
= [ f(x + h) – f(x) ] / h
The preceding equation is also the formula for the difference quotient. If h tends to 0, the secant becomes the curve’s tangent. In other words, if h tends to zero, the difference quotient formula will yield the derivative of the given function.
The steps for calculating the difference quotient for f(x) are as follows:
- Replace f(x) with f(x + h).
- Subtraction of f(x) from f(x + h); f(x + h) – f (x)
- Remove the brackets to simplify the expression.
- Divide this simplified equation by h. The resulting value is the difference quotient of the supplied function.
Difference quotient calculator with fractions
Method 1
Finding the difference quotient when your function is a fraction might be difficult, but there is a simple solution!
To begin, you’ll need to calculate the difference quotient in the same manner you would for any other non-fraction function. The tough part about these issues is that if you do this, you’ll wind up with “small fractions” inside of a “large fraction.”
To get rid of the tiny fractions, multiply the numerator and denominator of the big fraction by the common denominator of the little fractions. That manner, the fractions will cancel out in a single, rapid step.
Read Also:Coterminal Angle Calculator Formula, Solved Examples, Step by Step
Then all you have to do is multiply out and simplify the numerator, and ideally cancel out a “h” from the numerator and denominator. When trying to get the difference quotient for a fraction, this method will always work.
Method 2
Let’s break out another difference quotient. Let’s try it again with f(x) = 9 over x minus 2. So we begin with the difference quotient formula, f(x+h) minus f(x) divided by h.
f(x+h) for this function is 9 over x plus h minus 2. 9 multiplied by x plus h minus 2. We have negative f(x), which is basically this, 9 over x minus 2 and everything over h.
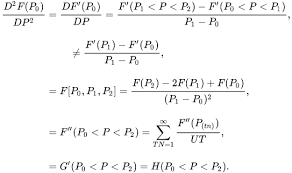
I’ve got a complicated fraction here, and I generally reduce these small denominators with a technique. The numerator and denominator of the large fraction multiplied by the least common denominator of the tiny fractions, yielding x + h minus 2, x minus 2. As a result, I double the top and bottom by that.
There will be a lot of cancelling in the following stage. All of this multiplied by 9 over x plus h minus 2 times. The x plus h minus 2’s will cancel, giving you 9 times x minus 2 negative. Now multiply this term by all of this, and the x minus 2’s will cancel, yielding 9 times x plus h minus 2. You won’t receive any cancellation in the denominator; instead, you’ll get h times these two men, so h times x plus h minus times x minus 2. Resist the need to double everything. It turns out that different quotients are employed in calculus, and it’s really preferable to leave this factored out of calculus.
Answer
Let’s make the numerator simpler. The left-hand phrase is 9x minus 18, while the right-hand term is minus 9x minus 9h plus 18, all over h times x plus h minus 2, times x minus 2. At this moment, we’re looking for cancellation. The 9x’s cancel, as do the 18’s. So negative 9h over h times x plus h minus 2 times x minus 2 equals minus 9h over h times x.
Finally, we can get rid of the h’s. As a result, we have a negative 9 over x plus h minus 2 times x minus 2. That concludes our response.
Difference quotient calculator at a point
Whether ΔP is infinitesimal or finite, there is (at least theoretically, in the case of the derivative) a point range with limits P (0.5) ΔP (depending on the orientation—ΔF(P), δF(P) or ∇F(P)):
Lower Boundary = LB; Upper Boundary = UB
Derivatives can be thought of as functions in and of themselves, with their own derivatives. As a result, each function is the home of successive degrees (“higher orders”) of derivation or differentiation. This characteristic applies to all difference quotients.
Because this sequencing necessitates comparable boundary splintering, it is feasible to divide the point range into smaller, equal-sized portions, each designated by an intermediary point (Pi), where LB = P0 and UB = P, the nth point, equaling the degree/order:
LB = P0 = P0 + 0Δ1P = Pń − (Ń-0)Δ1P;
P1 = P0 + 1Δ1P = Pń − (Ń-1)Δ1P;
P2 = P0 + 2Δ1P = Pń − (Ń-2)Δ1P;
P3 = P0 + 3Δ1P = Pń − (Ń-3)Δ1P;
↓ ↓ ↓ ↓
Pń-3 = P0 + (Ń-3)Δ1P = Pń − 3Δ1P;
Pń-2 = P0 + (Ń-2)Δ1P = Pń − 2Δ1P;
Pń-1 = P0 + (Ń-1)Δ1P = Pń − 1Δ1P;
UB = Pń-0 = P0 + (Ń-0)Δ1P = Pń − 0Δ1P = Pń;
ΔP = Δ1P = P1 − P0 = P2 − P1 = P3 − P2 = … = Pń − Pń-1;
ΔB = UB − LB = Pń − P0 = ΔńP = ŃΔ1P.
Difference quotient calculator square roots
The formula for a function’s difference quotient f (x). That is to say:
[f(x + h) – f(x)] / h
where (x, f(x)) and (x + h, f(x + h)) are any two points on the function of f’s graph (x). This formula calculates the slope of a line connecting any two points on the graph of f. The most practical application of this difference quotient is in determining derivatives. As h approaches zero, the limit of the difference quotient equals the derivative of the function f.
Derivative of f(x)
Lim h tends to 0 f(x+h) – f(x)/h
As a result, it is usually desired to be able to reduce a function’s difference quotient to the point where, if h = 0, there will not be a zero denominator.
Keeping this in mind, let’s look at functions that use radicals. The first step in determining the difference quotient of a radical function is the same as it is for determining the difference quotient of any function. To put it another way, we obtain f(x + h) and then input f(x) and f(x + h) into the difference quotient formula.
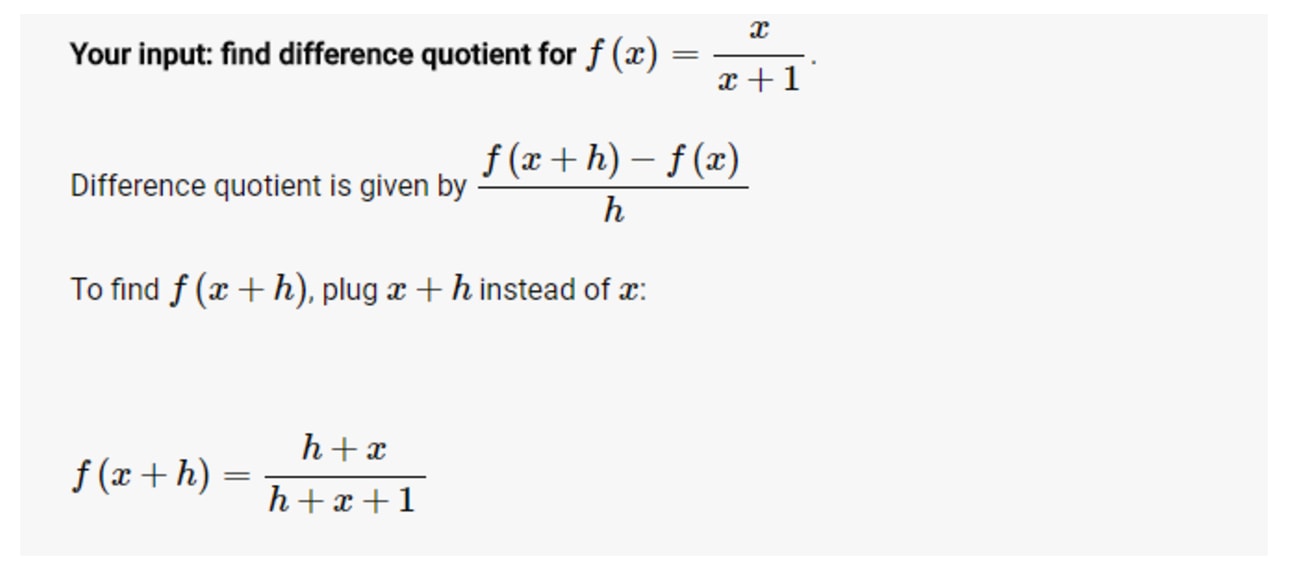
Consider the most basic radical function, f(x) = 1. (x). The first step in determining the difference quotient for this function is to calculate f(x + h) by substituting x + h for x in f to obtain:
f(x + h) = √(x + h)
We next enter f(x) and f(x + h) into the difference quotient formula.
We get [(x + h) – (x)] / h as a result. That appears to be the limit, however if h = 0, there would be a zero denominator, so we really want to be able to carry it farther. We’ll have to think beyond the box!
Solution
Conjugates are used in the following stage in determining the difference quotient of radical functions. The conjugate of the formula a + b is a – b, and it is also true that (a + b)(a – b) = a2 – b2 will significantly assist us in simplifying this difference quotient.
When it appears that we will be unable to simplify the difference quotient with radicals, the next step is to identify the numerator’s conjugate and multiply both the numerator and denominator by that conjugate.
In our case, the numerator is √(x + h) – √(x), hence the conjugate is √(x + h) – √(x). Take a look at what occurs when we multiply the numerator and denominator by this conjugate.
We obtain 1 / [root(x + h) + root(x)] after all the simplifications, and we see that if h = 0, we don’t have a zero denominator.
Difference quotient calculator f(x)-f(a)/x-a
Do so in the following manner:
f(x)−f(a)/x−a =1/x−1/a /x−a= (a−x) /ax(x−a)=−1/xa
You appear to be perplexed about how to assess or what f(a) for a given function entails. The function f is a rule that describes what happens to item x when the rule f is applied. This is spelled f (x). For instance, f(x)=1/x indicates that the rule f is to discover the reciprocal of whatever is supplied to it. f(5)=1/5, f(-1/2)= -2, f(a)=1/a, and so on.
Difference quotient calculator examples
Example 1
Using the difference quotient calculator, calculate the difference quotient for f(x) = 2x + 5.
Solution:
Given that f(x) = 2x + 5
f(x + h) = 2(x + h) + 5
= 2x + 2h + 5
Difference quotient = [ f(x + h) – f(x) ] / h
= [2x + 2h + 5 – 2x – 5] / h
= 2h / h
= 2
Example 2
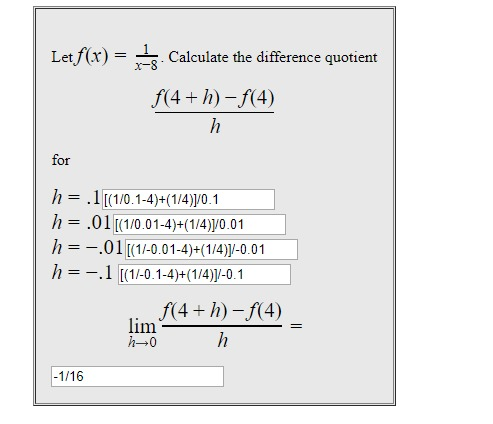
Using the difference quotient calculator, calculate the difference quotient for f(x) = (x2 – 1) / 3.
Solution:
Given that f(x) = (x2 – 1) / 3
Then, f(x + h) = [(x + h)2 – 1] / 3
Then, = [x2 + 2xh + h2 – 1] / 3
Difference quotient = [ f(x + h) – f(x) ] / h
Then, = [(x2 + 2xh + h2 – 1) – (x2 – 1) ] / 3h
Then, = (h + 2x)/ 3
Now, use the difference quotient calculator to calculate the difference quotient for the following:
f(x) = 5×2 + 7
f(x) = – 4x + 9
Example 3
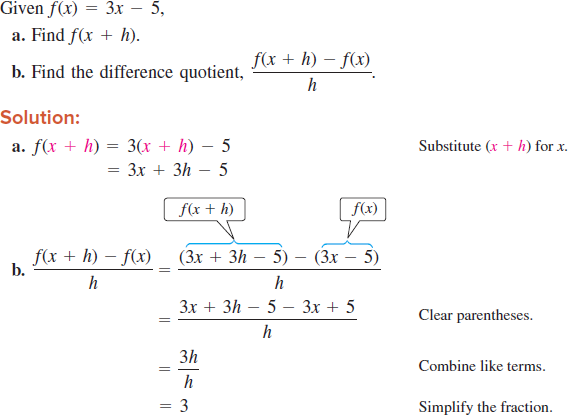
Evaluate the difference quotient of a function (f) defined by
F(x) = x^2 + 4
Solution:
Formula to find Difference Quotient is:
f(x) = f (x + h) – f (x) / h
To find f(x + h), put x + h instead of x:
f (x + h) = (x + h)^2 + 4
Then,
f(x) = f (x + h) – f (x) / h
Then, f(x) = ((x + h)^2 + 4) – (x^2 + 4)
Then, = h + 2x
As a result, the difference quotient for f (x) = x^2 + 4 equals h + 2x. It may be found by entering these numbers into the difference quotient calculator.
An online Derivative Calculator, on the other hand, will allow you to compute the derivative of a function with respect to a particular variable.
Difference quotient calculator Symmetric
In mathematics, a difference quotient is a formula that gives an estimate of a function’s derivative. There are two types of difference quotients: one-sided difference quotients and symmetric difference quotients. They are all connected, and one provides a better approximation than the others because of this connection.
The symmetric derivative is a mathematical procedure that generalises the ordinary derivative. It is defined as follows:
Lim h tends to 0 f(x+h) – f(x)/h
The equation below the limit is also known as the symmetric difference quotient. If a function’s symmetric derivative exists at point x, it is said to be symmetrically differentiable at that point.
If a function is differentiable (in the ordinary sense) at a point, it is also symmetrically differentiable, but not vice versa. The absolute value function f(x) = |x|, which is not differentiable at x = 0, but is symmetrically differentiable here with symmetric derivative 0, is a well-known counterexample. The symmetric difference quotient provides a better numerical estimate of the derivative than the standard difference quotient for differentiable functions.
If both the left and right derivatives exist at a given location, the symmetric derivative is the arithmetic mean of the latter two.
For the symmetric derivative, neither Rolle’s theorem nor the mean value theorem apply; several comparable but weaker claims have been proven.
Difference quotient calculator graph
The slope of the secant line passing through two points on the graph of f computed using this formula. These are the x-coordinate positions x and x + h. The derivative defined using the difference quotient. First, insert (x + h) anywhere you see an x into your function. Once you’ve determined f (x + h), enter your numbers into the difference quotient formula and simplify from there. The subtraction sign used in the third step to remove the parentheses and simplify the difference quotient.
You’ll see in the technical definition of the difference quotient that the slope we’re computing is for the secant line. A secant line is simply any line that connects two points on a curve. On our x-axis, we label these two locations as x and (x +h). Because we are working with a function, these points are labelled on our y-axis as f (x) and f (x + h), respectively.
In layman’s words, the difference quotient assists us in determining the slope of a curve. We cannot apply the standard formula of: in the case of a curve.
As a result, we must employ the difference quotient formula.
y1-y2/x1-x2
Some frequently asked questions
How do you find the difference quotient and simplify on a calculator?
The following are the methods we use to determine the difference quotient:
f(x+h)-f(x)/h
- Insert x + h into the function f and simplify to get f(x + h).
- Now that you’ve calculated f(x + h), calculate f(x + h) – f(x) by inserting in f(x + h) and f(x) and simplifying.
- Fill in your step 2 result for the numerator in the difference quotient and simplify it.
What is a difference quotient example?
Using the difference quotient calculator, calculate the difference quotient for f(x) = 2x + 5.
Solution:
Given that f(x) = 2x + 5
f(x + h) = 2(x + h) + 5
= 2x + 2h + 5
Difference quotient = [ f(x + h) – f(x) ] / h
= [2x + 2h + 5 – 2x – 5] / h
= 2h / h
= 2
Is the difference quotient the same as the derivative?
The difference quotient is a measure of the function’s average rate of change across an interval (in this case, an interval of length h). The instantaneous rate of change is thus the limit of the difference quotient (i.e., the derivative).
What is the difference quotient calculator?
However, the Difference Quotient Calculator is a free online calculator. Moreover, a difference quotient calculator online can help you calculate the difference quotient for a given function. Then, this difference of quotient calculator performs stepwise calculations to determine the slope of a secant line that connects two locations
Why is the difference quotient important for calculus?
We may compute the slope of secant lines using the difference quotient. A secant line is similar to a tangent line, except it passes through at least two points on a function. Finally, with some term cancellation, we may arrive at the definition of the difference quotient.
Who invented the difference quotient?
The difference quotient between two points as close together as possible, indicating the rate of change of a function at a particular point. Isaac Newton coined and defined the term “difference quotient”.
How do you calculate in the difference quotient calculator?
- First, navigate to Cuemath’s online difference quotient calculator.
- Then, enter the function into the difference quotient calculator’s supplied input box.
- Then, press the “Calculate” button to compute the difference quotient of the specified function.
- Atlast, click the “Reset” button to clear the box and input new values.
Why is the symmetric difference quotient more accurate?
The symmetric difference quotient provides a better numerical estimate of the derivative than the standard difference quotient for differentiable functions. If both the left and right derivatives exist at a given location, the symmetric derivative is the arithmetic mean of the latter two.
What is the difference in quotient calculus?
The Difference Quotient, often known as the, is an algebraic approach to the Derivative (dx. dy.). “The Four-Step Method.” It is a method for determining the slope of a line tangent to a function f(x) at a point (x) on the function that is continuous at that point (x).